Electromagnetic wave

In physics, an electromagnetic wave is a change, periodic in space and time, of an electric field E(r,t) and a magnetic field B(r,t). A stream of electromagnetic waves is referred to as electromagnetic radiation. Because an electric as well as a magnetic field is involved, the term electromagnetic (EM) is used, a contamination of electric and magnetic. Examples of EM waves in increasing wavelength are: gamma rays, X-rays, ultraviolet light, visible light, infrared, microwaves, and radio waves. All these waves propagate in vacuum with the same speed c, the speed of light. The speed of light in air (at standard temperature and pressure) is very close to the speed of light in vacuum (the refractive index of air, n, is 1.0002926, meaning that the speed of electromagnetic waves in air is c/n ≈ c).

Classically (non-quantum mechanically), EM radiation is produced by accelerating charges, for instance, by the oscillating charge in a radio antenna. Quantum mechanically, EM radiation is emitted whenever a system in an energetically high state (of energy E₂) makes a transition to a state of lower energy (E₁); during this transition a photon (light quantum) of energy proportional to the difference E₂ - E₁ > 0 is emitted. This is what happens in a fluorescent tube: mercury atoms are brought into an energetically high state by collisions with electrons, and upon subsequent falling down to their lowest energy state they emit photons.

Electromagnetic waves were predicted on theoretical grounds by James Clerk Maxwell in 1861 and first emitted and received in the laboratory by Heinrich Hertz a quarter century later. The first to see the applicability for communication purposes was the inventor of radiotelegraphy Guglielmo Marconi (around 1900). Later applications are radio, television, radar, cellular phones, gps, and all kinds of wireless applications, from remote control of television sets to internet access in public places.

Fig. 1. Electromagnetic wave. Electric component (red) in plane of drawing; magnetic component (blue) in orthogonal plane. Wave propagates to the right. The wavelength is λ.

Properties

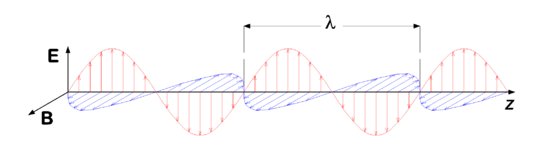
In figure 1 we see a snapshot (i.e., a picture at a certain point in time) of the magnetic and electric fields in adjacent points of space. In each point, the vector E is perpendicular to the vector B. The wave propagates to the right, along an axis which we conveniently refer to as z-axis. Both E and B are perpendicular to the propagation direction, which is expressed by stating that an electromagnetic wave is a transverse wave, in contrast to sound waves, which are longitudinal waves (i.e., air molecules vibrate parallel to the propagation direction of the sound).

Assume that the snapshot in figure 1 is taken at time t, then at a certain point z we see an arrow of certain length representing E(z,t) and also a vector B(z,t). At a point in time Δt later, the same values of E and B (same arrows) are seen at z + c Δt. The arrows seem to have propagated to the right with a speed c.

The time t is fixed and the position z varies in figure 1. Conversely, we can keep the position fixed and imagine what happens if time changes. Focus on a fixed point z, then in progressing time the two vectors E(z,t) and B(z,t) in the point z, grow to a maximum value, then shrink to zero, become negative, go to a minimum value, and grow again, passing through zero on their way to the same maximum value. This cycle is repeated indefinitely. When we now plot E and B in the fixed point z as a function of time t, we see the same type (sine-type) function as in figure 1. The number of times per second that the vectors go through a full cycle is the frequency of the electromagnetic wave.

Periodicity in space means that the EM wave is repeated after a certain distance. This distance, the wavelength is traditionally designated by λ, see figure 1. If we go at a fixed time a distance λ to the right or to the left we encounter the very same fields E and B.

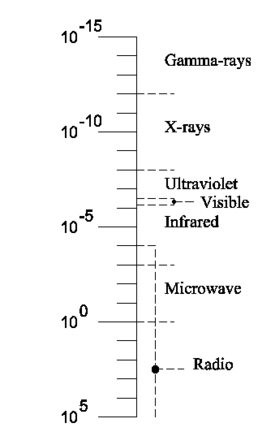
Basically, the only property distinguishing different kinds of EM waves, is their wavelength, see figure 2. Note the enormous span in wavelengths, from one trillionth of a millimeter for gamma-rays (radioactive rays) up to the VLF (very low frequency) radio waves of about 100 kilometer.

Frequency of electromagnetic waves

Often EM waves are characterized by their frequency ν, instead of their wavelength λ. If the EM field goes through ν full cycles in a second, where ν is a positive integral number, the field has a frequency of ν Hz (hertz). The speed of propagation of the EM waves being c, in 1/ν seconds the wave propagates a distance c × (1/ν) meter (according to the formula: distance traveled is speed times traveling time). The distance covered in 1/ν seconds is by definition the wavelength λ:

Fig. 2. Schematic overview of the electromagnetic spectrum. Vertical axis: wavelengths in meter. Examples: infrared extends from 8 ·10⁻⁷ to 10⁻³ m; radio from 10⁻⁴ to about 10⁴ m.

$\lambda ={\frac {c}{\nu }}\quad \Longrightarrow \quad \nu ={\frac {c}{\lambda }}$

If we express c in m/s then λ is obtained in m. To convert quickly from wavelength to frequency we can approximate c by 3·10⁸ m/s.

As was pointed out above, the wavelengths of the various parts of the EM spectrum differ many orders of magnitude. Furthermore, the sources of the radiations, the interactions with matter, and the detectors employed differ widely, too. So, it is not surprising that in the past different parts of the spectrum were discovered at different times and that electromagnetic radiation of different wavelengths is called by different names. In the table and in figure 2 some illustrative values are given for several kinds of EM waves, together with their names.

TABLE

Some typical values of:  wavelength (λ), frequency (ν = c/λ), photon energy (hν), cycle time (T = 1/ν), and inverse wavelength [1/(100⋅λ)].
EM wave	λ  (m)	ν  (1/s)	hν  (J)	T  (s)	1/λ  (cm−1)
γ-rays	1.00⋅10−14	3.00⋅1022	1.98⋅10−11	3.33⋅10−23	1.00⋅1012
X-rays	5.00⋅10−10	6.00⋅1017	3.96⋅10−16	1.67⋅10−18	2.00⋅107
Ultraviolet	2.00⋅10−7	1.50⋅1015	9.90⋅10−19	6.67⋅10−16	5.00⋅104
Visible	6.00⋅10−7	5.00⋅1014	3.30⋅10−19	2.00⋅10−15	1.67⋅104
Infrared	5.00⋅10−6	6.00⋅1013	3.96⋅10−20	1.67⋅10−14	2.00⋅103
Microwave	1.00⋅10−2	3.00⋅1010	1.98⋅10−23	3.33⋅10−11	1.00
Radio	1.00⋅102	3.00⋅106	1.98⋅10−27	3.33⋅10−7	1.00⋅10−4

Monochromatic linearly polarized waves

The wave depicted in figure 1 is monochromatic, i.e., it is characterized by a single wavelength (monochromatic means "of one color". In the visible region, different wavelengths correspond to light of different colors). It is known that EM waves can be linearly superimposed, which is due to the fact that they are solutions of a linear partial differential equation, the wave equation (see next section). A linear superposition of waves is a solution of the same wave equation as the waves themselves. Such a superposition is also an electromagnetic wave (a propagating periodic EM field). If waves of different wavelengths are superimposed, then a non-monochromatic wave is obtained (the term multi-chromatic wave would be apt, but is never used). By means of Fourier analysis a non-monochromatic wave can be decomposed into its monochromatic components.

The electric field vectors in figure 1 are all in one plane, this is the plane of polarization, and a wave with one fixed polarization plane, is called linearly polarized.

The radiation of many lasers is monochromatic and linearly polarized (at least to a very good approximation).

Relation to Maxwell's equations

In this section it will be shown that the electromagnetic wave depicted in figure 1 is a solution of the Maxwell equations in the vacuum.

We assume that at some distance away from the source of EM waves (a radio transmitter, a laser, gamma radiating nuclei, etc.), there is no charge density ρ and no current density J. For that region of space, the microscopic (vacuum) Maxwell equations become (in SI units):

${\boldsymbol {\nabla }}\cdot \mathbf {B} =0,\qquad {\boldsymbol {\nabla }}\cdot \mathbf {E} =0,$

and

${\boldsymbol {\nabla }}\times \mathbf {B} ={\frac {1}{c^{2}}}{\frac {\partial \mathbf {E} }{\partial t}},\qquad {\boldsymbol {\nabla }}\times \mathbf {E} =-{\frac {\partial \mathbf {B} }{\partial t}}.$

Apply to the last Maxwell equation the following relation, known from vector analysis and valid for any (differentiable) vector field,

${\boldsymbol {\nabla }}\times ({\boldsymbol {\nabla }}\times \mathbf {E} )={\boldsymbol {\nabla }}({\boldsymbol {\nabla }}\cdot \mathbf {E} )-{\boldsymbol {\nabla }}^{2}\mathbf {E}$

and use that ∇ · E = 0, then E satisfies the wave equation,

${\boldsymbol {\nabla }}^{2}\mathbf {E} ={\frac {\partial ({\boldsymbol {\nabla }}\times \mathbf {B} )}{\partial t}}={\frac {1}{c^{2}}}{\frac {\partial ^{2}\mathbf {E} }{\partial t^{2}}}.$

Note that the displacement current (time derivative of E) is essential in this equation, if it were absent (zero), the field E would be a static, time-independent, electric field, and there would be no waves.

In the very same way we derive a wave equation for B,

${\boldsymbol {\nabla }}^{2}\mathbf {B} ={\frac {1}{c^{2}}}{\frac {\partial ^{2}\mathbf {B} }{\partial t^{2}}}.$

Observe that E and B are related by the third and fourth Maxwell equation, which express the fact that a displacement current causes a magnetic field, and a changing magnetic field causes an electric field (Faraday's law of induction), respectively. So a time-dependent electric field that is not associated with a time-dependent magnetic field cannot exist, and conversely. Indeed, in special relativity E and c B can be transformed into one another by a Lorentz transformation of the electromagnetic field tensor, which shows their close relationship.

The wave equation is without doubt the most widely studied differential equation in mathematical physics. In figure 1 the electric field depicts a particular solution, with special initial and boundary conditions. The snapshot that is depicted has the analytic form

$\mathbf {E} (\mathbf {r} ,t)=\mathbf {e} _{x}E_{0}\sin {\big [}k(z-ct){\big ]}\quad {\hbox{with}}\quad k\equiv {\frac {2\pi }{\lambda }}.$

The snapshot is taken at $\scriptstyle t=2\pi n/(ck)$ for some arbitrary integer n. We assumed here that the direction of E defines the direction of the x-axis with unit vector e_x along this axis. The quantity E₀ is the amplitude of the wave. Insertion of this expression in in the left hand side of the wave equation for E gives

${\boldsymbol {\nabla }}^{2}\mathbf {E} =\mathbf {e} _{x}E_{0}{\frac {\partial ^{2}\sin {\big [}k(z-ct){\big ]}}{\partial z^{2}}}=-k^{2}\mathbf {e} _{x}E_{0}\sin {\big [}k(z-ct){\big ]}.$

Insertion of this expression in in the right hand side of the wave equation for E gives

${\frac {\mathbf {e} _{x}E_{0}}{c^{2}}}{\frac {\partial ^{2}\sin {\big [}k(z-ct){\big ]}}{\partial t^{2}}}=-{\frac {c^{2}\,k^{2}}{c^{2}}}\mathbf {e} _{x}E_{0}\sin {\big [}k(z-ct){\big ]}=-k^{2}\mathbf {e} _{x}E_{0}\sin {\big [}k(z-ct){\big ]},$

so that it follows that the special solution, depicted in figure 1, is indeed a solution of the wave equation for E.

We could now proceed in the very same way and solve the wave equation for B, but then we could easily overlook the relation between the two fields. So we rather substitute the solution for E into the fourth Maxwell equation and use the definition of curl as a determinant,

${\begin{vmatrix}\mathbf {e} _{x}&\quad \mathbf {e} _{y}&\mathbf {e} _{z}\\{\frac {\partial }{\partial x}}&\quad {\frac {\partial }{\partial y}}&{\frac {\partial }{\partial z}}\\E_{0}\sin {\big [}k(z-ct){\big ]}&\quad 0&\quad 0\\\end{vmatrix}}=k\mathbf {e} _{y}E_{0}\cos {\big [}k(z-ct){\big ]}=-{\frac {\partial \mathbf {B} }{\partial t}}.$

It is easy to see that

$\mathbf {B} (\mathbf {r} ,t)=\mathbf {e} _{y}B_{0}\sin {\big [}k(z-ct){\big ]}\quad {\hbox{with}}\quad B_{0}\equiv {\frac {E_{0}}{c}}$

is a solution of this equation. It follows that E and B are perpendicular (along the x-axis and y-axis, respectively) and are in phase. That is, E and B are simultaneously zero and attain simultaneously their maximum and minimum. The fact that (in vacuum) the amplitude of B is a factor c smaller than that of E is due to the use of SI units, in which the amplitudes have different dimensions. In Gaussian units this is not the case and E₀ = B₀.

Energy and energy flow of electromagnetic field

In this section the following balance of power (energy per unit time) densities will be derived:

${\boldsymbol {\nabla }}\cdot \mathbf {S} +{\frac {\partial {\mathcal {E}}_{\textrm {Field}}}{\partial t}}=-{\mathcal {E}}_{\textrm {Joule}}$

where

${\begin{aligned}\mathbf {S} &=\mathbf {E} \times \mathbf {H} \equiv \mathbf {E} \times {\frac {1}{\mu _{0}}}\mathbf {B} \\{\mathcal {E}}_{\textrm {Field}}&={\frac {1}{2}}(\mathbf {E} \cdot \mathbf {D} +\mathbf {B} \cdot \mathbf {H} )\equiv {\frac {1}{2}}(\epsilon _{0}\mathbf {E} \cdot \mathbf {E} +{\frac {1}{\mu _{0}}}\mathbf {B} \cdot \mathbf {B} )\\{\mathcal {E}}_{\textrm {Joule}}&=\mathbf {E} \cdot \mathbf {J} \\\end{aligned}}$

The terms in this equation have dimension W/m³ (power per volume). The quantity $\scriptstyle -{\mathcal {E}}_{\textrm {Joule}}\,>\,0$ represents the rate at which energy is produced per unit volume by ordinary Joulean (resistance) heating. The quantity $\scriptstyle {\mathcal {E}}_{\textrm {Field}}$ is the energy density of the EM field and the time derivative is the rate of increase (power density). The vector S, Poynting's vector, is the power flux, the amount of energy crossing unit area perpendicular to the vector, per unit time.

Multiplying the terms by a volume ΔV and a time span Δt, both small enough that the terms in the equation may assumed to be constant over the volume and the time span, the equation represents the conservation of energy: the energy generated in ΔVΔt (the right hand side) is equal to the increase in energy in ΔV plus the net flow of energy leaving ΔV. Hence, when this equation is multiplied by ΔVΔt it is an equation of continuity for energy.

Derivation

Recall from elementary electricity theory the laws of Joule and Ohm . They state that the amount of energy W per unit time, produced by a conduction current I, is equal to

$W=I^{2}\,R=I\,V,\quad {\hbox{with}}\quad R,\,W>0,$

where R is the resistance and V a voltage difference.

Assuming that the current flows along z, we introduce the current density J_z, and using

$V=-E_{z}\Delta z\quad {\hbox{and}}\quad I=J_{z}\Delta x\Delta y$

we obtain

$W=-E_{z}J_{z}\,\Delta x\Delta y\Delta z.$

We could continue discussing the system with the small volume $\Delta x\Delta y\Delta z$. However, because all terms in the equations would be multiplied by the same volume, it is more convenient to consider densities and to divide out the volume. Nevertheless, we still refer to the system. Thus, we define

${\mathcal {E}}_{\textrm {Joule}}\equiv -{\frac {W}{\Delta x\Delta y\Delta z}}=\mathbf {E} \cdot \mathbf {J} <0.$

The negative quantity $\scriptstyle {\mathcal {E}}_{\textrm {Joule}}$ is the loss of energy of the system per unit time and per unit volume (according to Joule's and Ohm's laws). One may look upon the quantity $\scriptstyle -{\mathcal {E}}_{\textrm {Joule}}$ as the work (per unit time and unit volume) done by the Lorentz force $\scriptstyle q(\mathbf {v} \times \mathbf {B} +\mathbf {E} )$ on the moving particles constituting the current density J. Since $\scriptstyle \mathbf {v} \cdot (\mathbf {v} \times \mathbf {B} )=0$, this work depends only on the electric field E.

Apply one of Maxwell's equations:

$\mathbf {E} \cdot \mathbf {J} =\mathbf {E} \cdot \left(-\epsilon _{0}{\frac {\partial \mathbf {E} }{\partial t}}+{\frac {1}{\mu _{0}}}{\boldsymbol {\nabla }}\times \mathbf {B} \right)$

Use a rule known from vector analysis and apply another one of Maxwell's equations,

${\begin{aligned}\mathbf {E} \cdot ({\boldsymbol {\nabla }}\times \mathbf {B} )=&\mathbf {B} \cdot ({\boldsymbol {\nabla }}\times \mathbf {E} )-{\boldsymbol {\nabla }}\cdot (\mathbf {E} \times \mathbf {B} )\\=&-\mathbf {B} \cdot \left({\frac {\partial \mathbf {B} }{\partial t}}\right)-{\boldsymbol {\nabla }}\cdot (\mathbf {E} \times \mathbf {B} )\end{aligned}}$

Define

${\mathcal {E}}_{\textrm {Field}}\equiv {\frac {1}{2}}\left(\epsilon _{0}E^{2}+{\frac {1}{\mu _{0}}}B^{2}\right)={\frac {1}{2}}\left(\mathbf {E} \cdot \mathbf {D} +\mathbf {B} \cdot \mathbf {H} \right)$

with

$\mathbf {D} \equiv \epsilon _{0}\mathbf {E} \quad {\hbox{and}}\quad \mathbf {B} \equiv \mu _{0}\mathbf {H} ,$

where ε₀ is the electric constant and μ₀ the magnetic constant of the vacuum. Define also

$\mathbf {S} \equiv {\frac {1}{\mu _{0}}}\mathbf {E} \times \mathbf {B} =\mathbf {E} \times \mathbf {H}$

where S is the Poynting vector called after John Henry Poynting. This vector is perpendicular to the plane of E and B and by the right-hand rule, it points in the direction of propagation of the EM wave. The divergence of the Poynting vector is the energy flow associated with the electromagnetic wave, i.e., with the pair E(r,t) and B(r,t). By definition ∇·S gives the flow leaving the system and − ∇·S gives the flow entering the system. The total energy balance becomes

$-{\mathcal {E}}_{\textrm {Joule}}={\frac {\partial {\mathcal {E}}_{\textrm {Field}}}{\partial t}}+{\boldsymbol {\nabla }}\cdot \mathbf {S} .$

Here we have found an example of the conservation of energy, known as Poynting's theorem: The energy produced per unit time according to Joule's law $\scriptstyle -{\mathcal {E}}_{\textrm {Joule}}$ is equal to the rate in increase of the electromagnetic energy of the system $\scriptstyle {\mathcal {E}}_{\textrm {Field}}$, plus the flow of EM radiation ∇·S leaving the system.

If there is no current, J = 0, then

${\frac {\partial {\mathcal {E}}_{\textrm {Field}}}{\partial t}}=-{\boldsymbol {\nabla }}\cdot \mathbf {S} ,$

which is the continuity equation. The increase of field energy per unit time is the flow of radiation energy into the system.

Example

As an example we give an order-of-magnitude-calculation of an electromagnetic energy density. Consider to that end a radio station with a signal of strength P kW. We compute the energy density at a distance R from the station. First we must assume what the shape is of the waves emitted by the antenna, are they spherical or cylindrical? We choose the latter and call the cylinder height z. Further it is assumed that power density is homogeneous and that all power crosses the cylindrical walls, that is, power crossing the top and bottom of the cylinder is assumed to be zero. Also no absorption by the atmosphere or the Earth will occur. When a steady state is reached (some time after the beginning of the transmission), the time derivative of $\scriptstyle {\mathcal {E}}_{\mathrm {Field} }$ vanishes. The energy density at a distance R becomes constant in time,

${\mathcal {E}}_{\mathrm {Field} }(R)={\frac {P}{2\pi Rzc}}\quad \mathrm {J/m^{3}} ,$

where c is the speed of propagation of the radio signal (is speed of ligth ≈ 3·10⁸  m/s). To give a numerical example: P = 100 kW, R = 5 km (about 3 miles), z = 50 m, then $\scriptstyle {\mathcal {E}}_{\mathrm {Field} }$ = 2.1·10⁻¹⁰ J/m³.

Quantization of the electromagnetic field

Einstein postulated in 1905 that an electromagnetic field consists of energy parcels (light quanta, later called photons) of energy hν, where h is Planck's constant. In 1927 Paul A. M. Dirac was able to fit the photon concept into the framework of the new quantum mechanics. He applied a technique which is now generally called second quantization, although this term is somewhat of a misnomer for EM fields, because they are, after all, solutions of the classical Maxwell equations, and it is the first time that they are quantized.

Second quantization

Second quantization starts with an expansion of a field in a basis consisting of a complete set of functions. The coefficients multiplying the basis functions are then interpreted as operators and (anti)commutation relations between these new operators are imposed, commutation relations for bosons and anticommutation relations for fermions (nothing happens to the basis functions themselves). By doing this, the expanded field is converted into a fermion or boson operator field. The expansion coefficients have become creation and annihilation operators: a creation operator creates a particle in the corresponding basis function and an annihilation operator annihilates a particle in this function.

Fourier series

In the case of EM fields the required expansion is a Fourier series, that is, an expansion of the type (for a real vector field A):

$\mathbf {A} (\mathbf {r} ,t)=\sum _{\mathbf {k} }\left(\mathbf {a} _{k}(t)e^{i\mathbf {k} \cdot \mathbf {r} }+{\bar {\mathbf {a} }}_{k}(t)e^{-i\mathbf {k} \cdot \mathbf {r} }\right)$

where the bar indicates complex conjugation. Such an expansion, labeled by a discrete (countable) set of vectors k, is always possible when A satisfies periodic boundary conditions, i.e., A(r + p,t) = A(r,t) for some finite vector p. To impose such boundary conditions, it is common to consider EM waves as if they are in a virtual box of finite volume V. Waves on opposite walls of the box are enforced to have the same value (usually zero). Note that the waves are not restricted to the box: the box is replicated an infinite number of times in x, y, and z direction.

Vector potential

It would be possible to quantize the electric and magnetic field separately, however, it is more efficient to quantize the vector potential in the Coulomb gauge. Since E and B are simply related to the vector potential, they are then quantized as well.

The magnetic field B is a transverse field and hence can be written as

$\mathbf {B} ={\boldsymbol {\nabla }}\times \mathbf {A} ,$

in which appears the vector potential A. Also the electric field E is transverse, because earlier we assumed absence of charge distributions. The electric field E also follows from A,

$\mathbf {E} =-{\frac {\partial \mathbf {A} }{\partial t}}.$

The fact that E can be written this way is due to the choice of Coulomb gauge for A:

${\boldsymbol {\nabla }}\cdot \mathbf {A} =0.$

By definition, a choice of gauge does not affect any measurable properties (the best known example of a choice of gauge is the fixing of the zero of an electric potential, for instance at infinity). The Coulomb gauge makes A transverse as well, and clearly A is in the same plane as E. (The time differentiation does not affect direction.) So, the vector fields A, B, and E are in a plane perpendicular to the propagation direction and can be written in terms of e_x and e_y (in the definition of figure 1). It is more convenient to choose complex unit vectors:

$\mathbf {e} ^{(1)}\equiv {\frac {1}{\sqrt {2}}}(\mathbf {e} _{x}+i\mathbf {e} _{y})\quad {\hbox{and}}\quad \mathbf {e} ^{(-1)}\equiv {\frac {1}{\sqrt {2}}}(\mathbf {e} _{x}-i\mathbf {e} _{y})$

which are orthonormal,

$\mathbf {e} ^{(\mu )}\cdot {\bar {\mathbf {e} }}^{(\mu ')}=\delta _{\mu ,\mu '}\quad {\hbox{with}}\quad \mu ,\mu '=1,\,-1.$

The Fourier expansion of the vector potential reads

$\mathbf {A} (\mathbf {r} ,t)=\sum _{\mathbf {k} }\sum _{\mu =-1,1}\left(\mathbf {e} ^{(\mu )}(\mathbf {k} )a_{\mathbf {k} }^{(\mu )}(t)\,e^{i\mathbf {k} \cdot \mathbf {r} }+{\bar {\mathbf {e} }}^{(\mu )}(\mathbf {k} ){\bar {a}}_{\mathbf {k} }^{(\mu )}(t)\,e^{-i\mathbf {k} \cdot \mathbf {r} }\right).$

It is an easy matter to construct the corresponding expansions for E and B from this expansion (differentiate with respect to time, and take curl, respectively).

Classical energy

The electromagnetic energy density $\scriptstyle {\mathcal {E}}_{\mathrm {Field} }$, defined earlier in this article, can be expressed in terms of the Fourier coefficients. We define the total energy (classical Hamiltonian) by

$H=\iiint _{V}{\mathcal {E}}_{\mathrm {Field} }(\mathbf {r} ,t)\mathrm {d} ^{3}\mathbf {r} .$

The classical Hamiltonian in terms of Fourier coefficients takes the form

$H=V\epsilon _{0}\sum _{\mathbf {k} }\sum _{\mu =1,-1}\omega ^{2}{\big (}{\bar {a}}_{\mathbf {k} }^{(\mu )}(t)a_{\mathbf {k} }^{(\mu )}(t)+a_{\mathbf {k} }^{(\mu )}(t){\bar {a}}_{\mathbf {k} }^{(\mu )}(t){\big )}$

with

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega \equiv |\mathbf{k}|c = 2\pi \nu = 2\pi \frac{c}{\lambda} }

and ε₀ is the electric constant. The two terms in the summand of H are identical (factors commute) and may be summed. However, after quantization (interpretation of the expansion coefficients as operators) the factors do no longer commute and according to quantum mechanical rules one must depart from the symmetrized classical Hamiltonian.

Quantization

The best known example of quantization is the replacement of the (time-dependent) linear momentum of a particle by the rule Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle \mathbf{p}(t) \rightarrow -i\hbar\boldsymbol{\nabla}} . Note that Planck's constant is introduced and that time disappears (in the so-called Schrödinger picture).

Here we do something similar and apply the quantization rules:

${\begin{aligned}a_{\mathbf {k} }^{(\mu )}(t)\,&\rightarrow \,{\sqrt {\frac {\hbar }{2\omega V\epsilon _{0}}}}\,a^{(\mu )}(\mathbf {k} )\\{\bar {a}}_{\mathbf {k} }^{(\mu )}(t)\,&\rightarrow \,{\sqrt {\frac {\hbar }{2\omega V\epsilon _{0}}}}\,{a^{\dagger }}^{(\mu )}(\mathbf {k} )\\\end{aligned}}$

subject to the boson commutation relations

${\begin{aligned}{\big [}a^{(\mu )}(\mathbf {k} ),\,a^{(\mu ')}(\mathbf {k} '){\big ]}&=0\\{\big [}{a^{\dagger }}^{(\mu )}(\mathbf {k} ),\,{a^{\dagger }}^{(\mu ')}(\mathbf {k} '){\big ]}&=0\\{\big [}a^{(\mu )}(\mathbf {k} ),\,{a^{\dagger }}^{(\mu ')}(\mathbf {k} '){\big ]}&=\delta _{\mathbf {k} ,\mathbf {k} '}\delta _{\mu ,\mu '}.\end{aligned}}$

The square brackets indicate a commutator, defined by

${\big [}A,B{\big ]}\equiv AB-BA$

for any two quantum mechanical operators A and B.

Hamiltonian

Substitution of the operators into the classical Hamiltonian gives the Hamilton operator of the EM field

${\begin{aligned}H&={\frac {1}{2}}\sum _{\mathbf {k} ,\mu =-1,1}\hbar \omega {\Big (}{a^{\dagger }}^{(\mu )}(\mathbf {k} )\,a^{(\mu )}(\mathbf {k} )+a^{(\mu )}(\mathbf {k} )\,{a^{\dagger }}^{(\mu )}(\mathbf {k} ){\Big )}\\&=\sum _{\mathbf {k} ,\mu }\hbar \omega {\Big (}{a^{\dagger }}^{(\mu )}(\mathbf {k} )a^{(\mu )}(\mathbf {k} )+{\frac {1}{2}}{\Big )}\end{aligned}}$

By the use of the commutation relations the second line follows from the first. Note that $\hbar \omega =h\nu =\hbar c|\mathbf {k} |$ , which is the well-known Einstein expression for photon energy. Remember that ω depends on k, even though it is not explicit in the notation. The notation ω(k) could have been introduced, but is not common.

Digression: harmonic oscillator

The second quantized treatment of the one-dimensional quantum harmonic oscillator is a well-known topic in quantum mechanical courses. We digress and say a few words about it. The harmonic oscillator Hamiltonian has the form

$H=\hbar \omega {\big (}a^{\dagger }a+{\frac {1}{2}}{\big )}$

where ω ≡ 2πν is the fundamental frequency of the oscillator. The ground state of the oscillator is designated by | 0 > and is referred to as vacuum state. It can be shown that $\scriptstyle a^{\dagger }$ is an excitation operator, it excites from an n fold excited state to an n+1 fold excited state:

$a^{\dagger }|n\rangle =|n+1\rangle {\sqrt {n+1}}\quad {\hbox{in particular}}\quad a^{\dagger }|0\rangle =|1\rangle \quad {\hbox{and}}\quad (a^{\dagger })^{n}|0\rangle \propto |n\rangle .$

Since harmonic oscillator energies are equidistant, the n-fold excited state | n > can be looked upon as a single state containing n particles (sometimes called vibrons) all of energy hν. These particles are bosons. For obvious reason the excitation operator $a^{\dagger }$ is called a creation operator.

From the commutation relation follows that the Hermitian adjoint $\scriptstyle a$ de-excites:

$a|n\rangle =|n-1\rangle {\sqrt {n}}\quad {\hbox{in particular}}\quad a|0\rangle \propto 0\rightarrow a|0\rangle =0,$

because a function times the number 0 is the zero function. For obvious reason the de-excitation operator $\,a$ is called an annihilation operator.

Suppose now we have a number of non-interacting (independent) one-dimensional harmonic oscillators, each with its own fundamental frequency ω_i. Because the oscillators are independent, the Hamiltonian is a simple sum:

$H=\sum _{i}\hbar \omega _{i}{\Big (}a^{\dagger }(i)a(i)+{\frac {1}{2}}{\Big )}.$

Making the substitution

$i\rightarrow (\mathbf {k} ,\mu )$

we see that the Hamiltonian of the EM field can be looked upon as a Hamiltonian of independent oscillators of energy ω = |k| c and oscillating along direction e^(μ) with μ=1,−1.

End digression; back to EM field

The quantized EM field has a vacuum (no photons) state | 0 >. The application of, say,

${\big (}{a^{\dagger }}^{(\mu )}(\mathbf {k} ){\big )}^{m}\,{\big (}{a^{\dagger }}^{(\mu ')}(\mathbf {k} '){\big )}^{n}\,|0\rangle \propto {\Big |}m^{(\mu )}(\mathbf {k} ),\,n^{(\mu ')}(\mathbf {k} ')\,{\Big \rangle },$

gives a quantum state of m photons in mode (μ, k) and n photons in mode (μ', k'). We use the proportionality symbol because the state on the right-hand is not normalized to unity.

We can shift the zero of energy and rewrite the Hamiltonian as

$H=\sum _{\mathbf {k} ,\mu }\hbar \omega N^{(\mu )}(\mathbf {k} )\quad {\hbox{with}}\quad N^{(\mu )}(\mathbf {k} )\equiv {a^{\dagger }}^{(\mu )}(\mathbf {k} )a^{(\mu )}(\mathbf {k} )$

The operator $N^{(\mu )}(\mathbf {k} )$ is the number operator. When acting on a quantum mechanical photon state, it returns the number of photons in mode (μ, k). Such a photon state is an eigenstate of the number operator. This is why the formalism described here, is often referred to as the occupation number representation.

Example

In an earlier example we introduced a radio station and calculated the electromagnetic energy density that the station creates in its environment; at 5 km from the station this was 2.1·10⁻¹⁰ J/m³. Let us now see if we need quantum mechanics to describe the broadcasting of this station.

The classical approximation to EM radiation is good when the number of photons is much larger than unity in the volume

$\left({\frac {\lambda }{2\pi }}\right)^{3},$

where λ is the length of the radio waves. In that case quantum fluctuations are negligible and cannot be heard.

Suppose the radio station broadcasts at ν = 100 MHz, then it is sending out photons with an energy content of νh = 1·10⁸× 6.6·10⁻³⁴ = 6.6·10⁻²⁶ J, where h is Planck's constant. The wavelength of the station is λ = c/ν = 3 m, so that λ/(2π) = 48 cm and the volume is 0.111 m³. The energy content of this volume element is 2.1·10⁻¹⁰ × 0.111 = 2.3 ·10⁻¹¹ J, which amounts to

3.5 ·10¹² photons per $\left({\frac {\lambda }{2\pi }}\right)^{3}$

Obviously, 3.5 ·10¹² is much larger than one and hence quantum effects do not play a role; the waves emitted by this station are well into the classical limit, even when it plays non-classical music, for instance of Led Zepplin.

External link

ISO 21348 Definitions of Solar Irradiance Spectral Categories