Relative permeability

(PD) Image: John R. Brews
Infinitesimal current elements in two closed current-carrying loops

In physics, in particular in magnetostatics, the relative permeability is an intrinsic property of a magnetic material. It is usually denoted by μ_r. For simple magnetic materials, using SI units, μ_r is related to the proportionality constant between the magnetic flux density B and the magnetic field H, namely B = μ_r μ₀ H, where μ₀ is the magnetic constant. The relative permeability describes the ease by which a magnetic medium may be magnetized.

A related quantity is the magnetic susceptibility, denoted by χ_m, related to the magnetic permeability in SI units by:

$\mu _{r}=1+\chi _{m}\ .\ \mathrm {SI\ units} \ .$

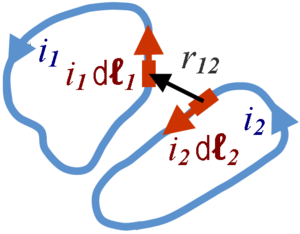
The Ampère force exerted between infinitesimal current elements from two loops carrying currents I_i and I_j and immersed in a magnetic medium with relative permeability μ_r is changed by a factor μ_r. The infinitesimal current elements have vector components I_idℓ_i and I_jdℓ_j where the incremental lengths are directed along the wire at the location of the element, and pointing in the direction of the current. Ampère's original force law is:

$d^{2}\mathbf {F_{ij}} =-d^{2}\mathbf {F_{ji}} =I_{i}I_{j}{\frac {\mu _{r}\mu _{0}}{4\pi }}{\frac {\mathbf {{\hat {u}}_{ij}} }{r_{ij}^{2}}}\left(2\ (d{\boldsymbol {\ell _{i}\cdot }}d{\boldsymbol {\ell _{j}}})-3(\mathbf {{\hat {u}}_{ij}\cdot } d{\boldsymbol {\ell _{i}}})(\mathbf {{\hat {u}}_{ij}\cdot } d{\boldsymbol {\ell _{j}}})\right)\ ,$

with û_ij a unit vector pointing along the line joining element j to element i and r_ij the length of this line. The force element is second order because it is a product of two infinitesimals. This force law leads to the same force between closed current loops as the more commonly used Grassmann's law:

$d^{2}\mathbf {F_{ij}} =I_{i}I_{j}{\frac {\mu _{r}\mu _{0}}{4\pi }}{\frac {1}{r_{ij}^{2}}}\left(\ (d{\boldsymbol {\ell _{i}\cdot }}d{\boldsymbol {\ell _{j}}})\mathbf {{\hat {u}}_{ij}} -(\mathbf {{\hat {u}}_{ij}\cdot } d{\boldsymbol {\ell _{i}}})d{\boldsymbol {\ell _{j}}}\right)\ ,$

which is obviously not symmetric under exchange of the indices i and j, and violates Newton's law of action and reaction. However, Grassmann's law is readily derived from the Biot-Savart law, and is consistent with space-time symmetry, unlike Ampère's law.

Empirically it is observed that the force may increase or decreases due to the presence of the magnetic medium, hence the relative permittivity μ_r may be greater than or less than 1. For simple media, if μ_r < 1, the medium is termed diamagnetic; if > 1 paramagnetic. Only classical vacuum has μ_r = 1 (exact). A typical diamagnetic susceptibility is about −10⁻⁵, while a typical paramagnetic susceptibility is about 10⁻⁴. It should be noted that the use of a constant as the relative permeability of a substance is an approximation, even for quantum vacuum. A more complete representation recognizes that all media exhibit departures from this approximation, in particular, a dependence on field strength, a dependence upon the rate of variation of the field in both time and space, and a dependence upon the direction of the field. In many materials these dependencies are slight; in others, like ferromagnets, they are pronounced.